Preliminary Results of Testing of Low-Tooth-Count Bevel Gears of a Novel Design, Part II

In the proposed approach, the tooth flanks of the gear, G, and the pinion, P, are generated by means of a desirable line of contact, LC_des. This means that the line of contact between the tooth flanks G and P is designed before the tooth flanks themselves are designed. Use of such an approach enables the gear designer designing bevel gears featuring the line of contact with the most favorable contact geometry. For more details on the contact geometry of surfaces (that is, of the tooth flanks G and P), the interested reader is referred to [1] and other advanced sources.

In the example, the desired line of contact, LC_des, between the tooth flanks of the gear, G, and the pinion,P, is a circular arc of a radius R_lc = 200 mm (Figure 1). The arc is entirely located within the plane of action, PA.  At the middle of the face width, the line of contact forms a spiral angle ψ_lc = 10º (not shown in Figure 1).

In a local reference system x_lcy_lcz_lc associated with the plane of action,PA, and centered at the center, O_lc, of the circular arc of the radius R_lc, the position vector of a point, r ^(lc) _des, of the desired line of contact, LC_des, can be analytically described by an expression in the form:

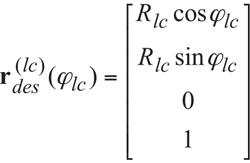
  (Equation 1)

where Φ_lc is the angular parameter of the desired line of contact, LC_des.

In a reference system X_pa Y_paZ_pa (not shown in Figure 2) associated with the plane of action,PA, and centered at the apex,A_pa, the position vector of a point,r ^(pa) _des, of the desired line of contact,LC_des, can be analytically described by an equation:

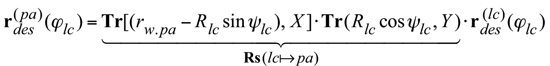
        (Equation 2)

where Tr[(r_w.pa –R_lc sin ψ_lc), X] and Tr[(R_lc cos ψ_lc), Y] are the standard operators of the translations along the coordinate axes X and Y, correspondingly. The standard formula [1] can be used for the calculation of these operators. Rs (lc  pa) is the operator of the resultant coordinate system transformation, that is, the operator of the transition from x_lcy_lcz_lc to X_pa Y_paZ_pa.  r_w.pa is the pitch (mid) diameter of the plane of action, and X is the shift of the reference system x_lcy_lcz_lc along the X axis of the reference system X_pa Y_paZ_pa.  In other words, r_w.pa and X are the coordinates of the origin O_lc in the reference system X_pa Y_paZ_pa. Ultimately, it can be shown that in the reference system X_pa Y_paZ_pa associated with the plane of action, the position vector of a point,r ^(pa) _des, can be rewritten in the following form:

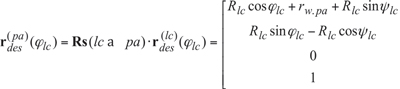
(Equation 3)

When the gears rotate, the desired line of contact, LC_des travels with respect to a reference system X_gY_gZ_g associated with the gear. Simultaneously, LC_des travels with respect to a reference system X_pY_pZ_p  associated with the pinion. Therefore, the gear tooth flank, G, can be viewed as a loci of consecutive positions of the line of contact, LC_des, in the reference system X_gY_gZ_g . Similarly, the pinion tooth flank, P, can be viewed as a loci of consecutive positions of the line of contact, LC_des, in the reference system X_pY_pZ_p.  To derive equations for the position vectors of a point of the gear tooth flank, r_g, and the pinion tooth flank, r_p, the operators, Rs (pa→g) and Rs (pa→p), of transition from the reference system X_paY_paZ_pa to the reference systems X_gY_gZ_g and X_pY_pZ_p are used:

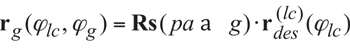
(Equation 4)

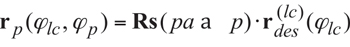
(Equation 5)

The operator of the resultant coordinate system transformation Rs (pa→g) is a function of the angle of rotation of the gear, Φ_g, and of the angular parameter, Φ_lc. The operator of the resultant coordinate system transformation Rs (pa→p) is a function of the angle of rotation of the pinion, Φ_p, and of the angular parameter Φ_lc.

The position vectors, r_g and r_p, are expressed in terms of Gaussian parameters Φ_lc and Φ_g of the gear tooth flank, G, and Gaussian parameters Φ_lc and Φ_p of the pinion tooth flank, P, correspondingly.

The operator Rs (pa→g) of the resultant coordinate system transformation can be represented in the form of the product of the operators of the rotations in [6]:

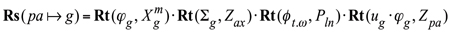   (Equation 6)

The operators of the rotations , , , and  are the standard operators of the linear transformations. For the calculation of the operators, standard formulae [1] can be used. Similarly, the operator of the resultant coordinate system transformation can be represented in the form of the product of the operators of the rotations:

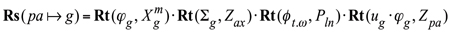  (Equation 7)

The operators of the rotations and  are also the standard operators of the linear transformations. For the calculation of the operators, standard formulae [1] can be used.

The developed mathematical models of the tooth flanks G and P can be used for the development of the CAD models of the gear and the pinion. Later on the CAD models are necessary for the manufacturing purposes of the gear and the pinion, that is, the CAD model can be converted to the corresponding G-code for a 5-axis NC machine for cutting the prototype gears. Another purpose of the CAD models is a more detailed investigation of the gearing by means of special FEA computer means. 

Additional Authors:
Vishnu Irigireddy, MS,Apex Tool Group, LLC, Lexington, South Carolina, USA. Phone: +803-808-6738 (off), 803-466-1087 (cell), e-mail: vishnu.irigireddy@apextoolgroup.com. Sandeep M. Vijayakar, Ph.D., advanced Numerical Solutions LLC. 3962 Brown Park Drive, Suite C, Hilliard OH 43026 USA, Phone: (614)771-4861 (614)771-4861, Fax: (614)453-8762, e-mail: sandeep@ansol.com. John Stewart, Apex Tool Group, LLC, Lexington, South Carolina, USA, Phone: +803-951-7559 (off), 803-800-4547 (cell), e-mail: John.Stewart@apextoolgroup.com. Ty P. Warner, P.E., TYKA Engineering, LLC, Eagle River, Wisconsin, USA, Phone: +218-341-9689 (cell), e-mail: tywarner@peoplepc.com