Vibration can be defined as the repetitive periodic motion of a machine and/or its components such as gear trains, gear drives, gear boxes, drive shafts, and couplings, just to name a few. A simple example is a weight suspended from a spring:
Some causes of vibration are gear tooth problems, bent shafts, imbalance of rotating parts, misalignment of shafts or bearings, damaged couplings, drive belts, loose parts, rubbing, flow (pumps and fans), and electrical problems. To determine or to analyze a vibration, two key elements must be considered. These are amplitude (how much) and frequency (how fast). There are a few ways to measure vibration:
Displacement: The total distance traveled by a moving part from one extreme to another. These measurements are taken in millimeters, or one thousandth of an inch (0.001 inch).
Velocity: As weight moves it has a speed, which is changing depending on what point in the motion we examine. At the top and bottom of the movement, the speed goes to zero, because the weight must stop and go back the other way. The highest, or peak velocity is chosen as the one we measure. The units are inches per second or peak (also written as IPS, or In/Sec).
Acceleration: Defined as the rate of change in velocity. The acceleration causes the speed or velocity to vary. The point of maximum acceleration is at the two extremes of the travel, because acceleration causes the velocity to come to zero, then turn and speed up in the opposite direction. The units for acceleration could be inches per second per second, but vibration experts have chosen to relate it to the acceleration caused by gravity. The acceleration due to earth's gravity is 32.2 feet per second, or 386 inches per second per second. The units for acceleration are g's peak. Frequency is the measurement of vibration that measures how fast the machine or its components are moving. The time for vibration to go through one cycle is the period shown in the Figure 2. It is measured in seconds or minutes.
The frequency (F) is the reciprocal of the period. Equation form becomes: F = 1/t. When the period is 0.01 minutes, for example, the frequency is: F = 1/0.01 = 100 cycles per minute.
Displacement: The displacement, x, is expressed as:
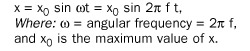
Velocity: Then by calculus, V is the derivative of displacement with respect to time, or:
![]()
Acceleration: Similarly, acceleration is the second derivative of displacement with respect to time, or:
![]()
In the conversion of amplitude units, there is a mathematical relationship between displacement, acceleration, and velocity. It is derived from the previous equations. To convert from displacement to velocity or acceleration, you must know the frequency, f.

Caution: Be aware that these conversion formulas only apply to a single pure sinusoidal waveform. For real vibration composed of several frequencies, this calculation can be difficult to do. However, modern data collection apparatus can automatically convert from one set of units to another. Equipment vibrates in complex fashion. We seldom see a simply sinusoidal vibration waveform. What we do is use Fast Fourier Technology (FFT) to break the complex waveforms into its sinusoidal vibration components. An experienced analyst looks at the resulting spectrum, waveform, and overall vibration data to determine the most likely cause of vibration.
In the next installment I will discuss gear tooth/bearing problems, along with when and why to schedule equipment repairs through replacement of bevel gears.




















